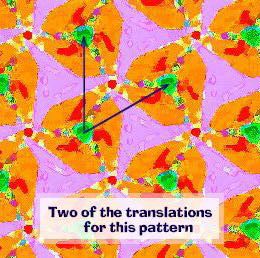
If you have a given repeating pattern, you can slide it along a certain direction a certain distance and it will fall back upon itself with all the patterns exactly matching. This symmetry is called a translation. The only patterns we'll consider are those with translation symmetries in at least two different directions.
In the example you see two arrows suggesting two of the translation symmetries. One points directly up, one up and to the right. There are more, of course, you could go up two units, or down one unit, or down and to the left. Indeed, there are infinitely many different translation symmetries for this and any other pattern that we consider.
Translations are just one kind of planar isometry. There are three others: rotations, reflections, and glide reflections.
Rotations

A rotation fixes one point in the plane and turns the rest of it some angle around that point. In general a rotation could be by any angle, but for patterns like we have, the angle has to divide 360°, and a little more analysis finds further restrictions. In fact, the angle of rotation can only be 180°, 120°, 90°, or 60°. The order of a rotation is the number of times it has to be performed to bring the plane back to where it started. So a 60°-rotation has order 6, a 90°-rotation has order 4, a 120°-rotation has order 3, and an 180°-rotation has order 2. A 180° rotation is also called a half turn.
In the example you see that a rotation of 90° preserves the pattern. And doing that rotation twice gives a rotation of 180° that preserves the pattern.
Reflections

A reflection fixes one line in the plane, called the axis of reflection, and exchanges points on one side of the axis with points on the other side of the axis at the same distance from the axis. In the example you see a diagonal axis in white. The double-ended red arrows are supposed to suggest the reflection. It's called a "reflection" because similar things happen with a reflection in a mirror.
In all our patterns, if there is one reflection, then the translations will guarantee that there are infinitely many reflections.
Glide reflections

The fourth kind of isometry, the glide reflection, is not nearly as easy to see as the other three. It's composed of a reflection across an axis and a translation along the axis. In the example, the axis of the glide reflection is drawn in magenta. The green arrows on either side of it suggest the glide reflection. If you reflect across the magenta axis, then translate along it (either up or down), one green arrow will fall onto another green arrow on the other side of the axis. Moreover, the whole pattern will fall onto itself if you apply the glide reflection, so this is a symmetry of the pattern.
Note that in the example, although there are translations and glide reflections that are symmetries, no rotations or reflections are symmetries.
Up to the table of contents
On to lattices
© 1997.
David E. Joyce
Department of Mathematics and Computer Science
Clark University
Worcester, MA 01610
The address of this file is http://aleph0.clarku.edu/~djoyce/wallpaper/